On the clique number of noisy random geometric graphs

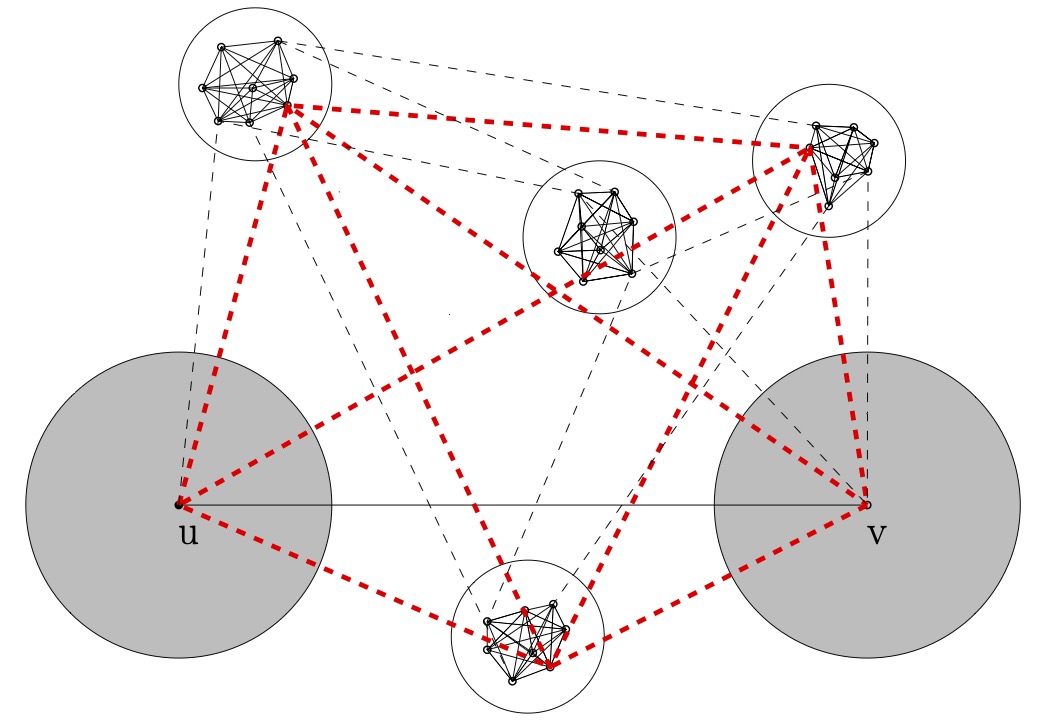
Let $G_n$ be a random geometric graph, and then for $\pp,\qqq \in [0,1)$ we construct a \emph{$(\pp,\qqq)$-perturbed noisy random geometric graph} $G_n^{\pp,\qqq}$ where each existing edge in $G_n$ is removed with probability $\pp$, while and each non-existent edge in $G_n$ is inserted with probability $\qqq$. We give asymptotically tight bounds on the clique number $\omega\left(G_n^{\pp,\qqq}\right)$ for several regimes of parameter.